20 replies to this topic

[ankur2061]

Dear All,

For fire case calculations for relief valves in the equation:

Q = 21000*F*A^0.82

A is the wetted surface area

Any of the learned forum members can probably give me an exact equation for calculating wetted surface area of a partially filled horizontal cylindrical vessel with 2:1 ellipsoidal heads.

I certainly know that there will be two components to the surface area. One will be the wetted surface area of the partially filled cylinder and the other will be the wetted surface area of the 2:! ellipsoidal head.

The total wetted surface will thus be

= Wetted surface area of partially filled cyllinder + 2*wetted surface area of partially filled 2:1 ellipsoidal head

Only thing I don't know are the exact equations and a pictorial representation for the angles and the wetted arc to be considered.

Art I would appreciate if you could throw some light on this.

Regards,
Ankur.

[Art Montemayor]

Ankur:

I created an Excel workbook some years ago to resolve this type of design problem. The name of the workbook is "Vessel Volumes" and it is mean to assist in determining this type resolution. There is a worksheet within that workbook that contains the surface area of varying sizes of vessel heads - 2:1 Ellipsoidal, ASME F&D, and Hemi-heads.

The partial volume of a horizontal vessel is easily obtained using this workbook and the partial head area can be estimated as a percentage of the the total - knowing the height of the liquid in the vessel (& heads).

You should be able to get within 10-15% accuracy by my method - and you should always make sure that it is on the conservative side. You should be able to find this workbook somewhere within our Forums. I have issued well over several hundred copies in the past and the amount downloaded from our Forums should be well over a thousand by now.

Hope this helps you out.

[joerd]

If you are mathematically inclined, there is an article by Richard Doane, in Chemical Engineering, December 2007, p. 56, "Accurate Wetted Areas For Partially Filled Vessels" that gives you an exact equation for ellipsoidal heads.

[Art Montemayor]

Ankur:

I forgot to mention that you can also use:

http://www.xcalcs.com/cgi-bin/tutti/x2calcs.cgi?l=en&b=i_3_9_0&d=i_3_9_0_1

as a means to obtain the information you need. Note that you have to go to different web pages for the different types of heads. Also, xcalcs if not too friendly in telling you all the sources, references, and derived equations that they use to come up with their solution.

[ankur2061]

Joerd,

Thanks for the info. By googling I did get a reference of the article but unfortunately I don't have access to the article.

I am trying to acquire it.

Regards,
Ankur.

[ankur2061]

Art,

Thanks for all the info. I have seen your excel workbook which is indeed very informative and I am trying to figure out the exact equation based on all the info provided till date by you and various other sources.

I will post the equation once I have worked through it for the benefit of the forum readers.

Regards,
Ankur.

[djack77494]

Ankur:

I forgot to mention that you can also use:
http://www.xcalcs.co...amp;d=i_3_9_0_1
as a means to obtain the information you need. Note that you have to go to different web pages for the different types of heads. Also, xcalcs if not too friendly in telling you all the sources, references, and derived equations that they use to come up with their solution.

Xcalcs may not be very friendly, but it's the only source I've found for calculating tilted partially filled (near) horizontal vessels. Least you think there is no need for such a caluclation, think about a floating oilo production platform. It may (will) tilt due to waves and other factors, and you may need to know properties of a partially filled vessel. Xcalcs is a good resource for this and other calcs.

[ankur2061]

Dear All,

After all due research and finding the necessary inputs, I have prepared a spreadsheet on the Wetted Surface Area of a horizontal vessel with 2:1 ellipsoidal head. Those who are genuinely interested may write to me on my e-mail ID and I will mail it to them.

e-mail ID: ankur_2061@hotmail.com

Regards,
Ankur.

[ankur2061]

Dear All,

The above post is 3 years old and there was really no point to open this post except that even today I receive mails asking me for the spreadsheet calculations for wetted surface area. As we all know that wetted surface area for vessels is an absolute must for doing fire case PSV sizing calculations for pressure vesssels acccording to API STD 521 and for atmospheric storage vessels emergency vents according to API STD 2000.

Today I am presenting the spreadsheet calculations as an attachment and`as a new year gift for the members of the forum.

Regards,
Ankur.

Attached Files

•  Vessel_2_is_to_1_Ellipsoidal_Vessel_.xls   138.5KB   1378 downloads

Edited by ankur2061, 01 January 2012 - 08:54 AM.

[ankur2061]

Dear All,

The original reference for the spreadsheet can be found at:]

http://webwormcpt.bl...e-area-for.html

Regards,
Ankur.

[SL89]

The equations from the Doane and Wong articles are not correct.
There was an article in Sept 2011 Chemical Engineering covering the same topic.
It can be seen in the attached graph that the Doane and Wong equations are incorrect - for example, surface area at 20% of liquid level will be less than 20% of the total surface area. The equations from those 2 articles indicate the opposite. The Xcalcs data is correct.

Attached Files

•  partial surface area comparison.pdf   108.32KB   546 downloads

[ankur2061]

SL89,

From the chart you have attached, the Doane and Wong articles give higher wetted surface area at lower liquid levels in a horiziontal vessel with 2:1 elllipsoidal heads.

The difference that I can see from the chart is that for a 20% liquid level in the vessel the Xcalc gives the surafce area to be 13% of the total surface area whereas the Doane method gives 24% of the total surface area.

My stand on this is that for doing a calculation for fire case relief valve which , I would take a chance with the higher surface area (11% higher) which would give me a higher vapor load for my relief valve rather than with a lower vapor load by considering a lower surface area based on the xCalcs.

The attached chart shows that for the lower filling of the vessel (below 50%) the difference between the Xcalcs and the Doane method does not exceed 11% for any of the lower fill levels. I think I can live with that.

And as mentioned in post# 7 xCalcs is not the most friendly source for providing backup information on what equations they have used to calculate the surface area of a partially filled horizontal vessel with 2:1 ellipsoidal heads. I would like to see the equations before i can really decide to switch to xCalcs for calculating the surface area of a partially filled vessel with 2:1 ellipsoidal heads.

Regards,
Ankur.

[big_al]

Thank you Ankur. Great Spreadsheet

[justboud]

Hi Everyone,

I know this is an old thread, but I've been trying to do partially filled heads for ASME 100-6 F&D heads and stumbled across this thread and signed up. After learning about xcalcs, I decided to use it to estimate surface areas at different liquid levels. However, I have deep concerns about the xcalc results. In the chart the SL89 posted, you can see that there is a "bump" in the curve for the xcalc result. But there's no dramatic change in the shape of the head that would warrant such a "bump". Like ankur, I tried googling an analytical solution to the problem and had no luck. But I was able to get results of my own using Google's SketchUp software.



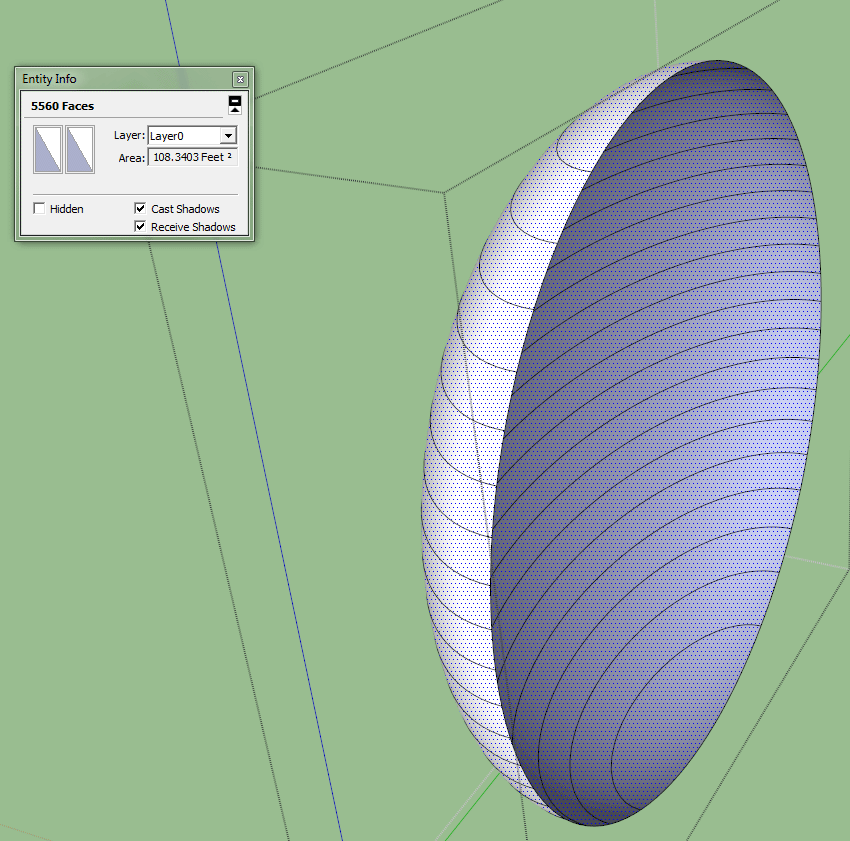
In google SketchUp, I made both a 10 ft diameter 2:1 Semi-elliptical head and a 100-6% ASME F&D head. Each head was then segmented into twenty 6" levels as shown in the screenshot above. You can also see that the 2:1 SE head total calculated surface area is 108.34 ft2 which corresponds well to the analytical solution of the total head surface area (1.084*D^2 = 1.084*10^2 = 108.4 ft2). SketchUp calculates the area of a given selection numerically and so I was able to get the surface area of each of the twenty segments. Using the chart the SL89 posted, I've added my results, which are different than xcalcs, Doane, and Wong.

Obviously, I'm quite biased, but I think my results are the best (mostly because I know how I got the results whereas the others I don't)!

Using a polynomial fit, I get the following equation for a 2:1 semi-elliptical head:
Ah/At = -0.4884*(h/D)^3 + 0.7341*(h/D)^2 + 0.7549*(h/D)

Also, for those interested, I get the following equation for an ASME 100-6% F&D head:
Ah/At = -0.8010*(h/D)^3 + 1.2051*(h/D)^2 + 0.5974*(h/d)

Where Ah is the partially filled surface area, At is the total head surface area, h is the liquid level, and D is the head diameter.

Attached Files

•  Head_SA.pdf   173.17KB   397 downloads

Edited by justboud, 12 October 2012 - 03:32 PM.

[kkala]

Thanks for the patient work ending to a simple formula on the wetted surface area of 2:1 ellipsoidal heads (and ASME 100-6% FandD heads) for horisontal drums partially filled. I have not tried to see how precise results (by justboud) are, yet these are closer to results by xcalcs for all liquid levels, as indicated in above attachement "Head_SA.pdf".

Edited by kkala, 14 October 2012 - 01:20 AM.

[Bobby Strain]

You can check any spreadsheet that you get with a thoroughly validated tool at www.Bobby-strain-group.com. The elliptical head wetted area is not exact, but it is close enough for fire exposed area. I'll take a look at the spreadsheets, too. I never found a means to directly calculate the partial area for elliptical head. To get the partial area of two heads, just set the length to 0. Let me know what you find. I recall that I simply use a linear relationship between the height and the surface area.

Bobby

Edited by Bobby Strain, 17 October 2012 - 04:12 PM.

[Pilesar]

justboud,

Your picture appears to be a simple revolved elliptical arc which may not adequately represent an ASME pressure vessel head. Can you get a volume calculation from Sketchup as a check? Baker Tankhead gives a reference volume equation at http://bakertankhead.../tank-heads.htm . See also the diagrams at that site. Is your shape different?

Heads have a straight flange section (skirt) which should be included when calculating surface area. Did you account for this in your calcs?

ASME Section VIII, Div 1, UG-32 states "An acceptable approximation of a 2:1 ellipsoidal head is one with a knuckle radius of 0.17D and spherical radius of 0.90D." Perhaps that shape would also make a useful check.

I would like to derive the surface area myself, but so far the math is eating my lunch. Would someone reveal the equations from the Sept 2011 Chemical Engineering article? I do not find a copy convenient to me.

Edited by Art Montemayor, 20 October 2012 - 10:14 AM.

[gegio1960]

Interesting discussion.
Anyhow, I don't think it's a must to have an extreme precision in calculating the wetted area of the heads: any good estimate with slight margin should be enough.
Kind regards

[justboud]

justboud,

Your picture appears to be a simple revolved elliptical arc which may not adequately represent an ASME pressure vessel head. Can you get a volume calculation from Sketchup as a check? Baker Tankhead gives a reference volume equation at http://bakertankhead.../tank-heads.htm . See also the diagrams at that site. Is your shape different?

Heads have a straight flange section (skirt) which should be included when calculating surface area. Did you account for this in your calcs?

ASME Section VIII, Div 1, UG-32 states "An acceptable approximation of a 2:1 ellipsoidal head is one with a knuckle radius of 0.17D and spherical radius of 0.90D." Perhaps that shape would also make a useful check.

I would like to derive the surface area myself, but so far the math is eating my lunch. Would someone reveal the equations from the Sept 2011 Chemical Engineering article? I do not find a copy convenient to me.

I was able to calculate volumes in Sketchup. For a 10' Dia. 2:1 SE head, I get a volume of 130.6 ft3 (977 gallons). The equation off the Baker Tankhead site is V = 0.000586 D3 where the diameter is in inches and the volume is in gallons. The Baker equation gives: V = 0.000586 * (10*12)^3 = 1013 gallons. So there's about a 3.6% error. However, looking at the Rules of Thumb for Mechanical Engineers, J. Edward Pope, the equation given is V = (pi*D^3)/24 = (pi*10^3)/24 = 130.9 ft3 or 979 gallons which is much closer to what I get. The Baker equation probably includes some straight flange where my calculations did not. For my calculations, I'm assuming that the length of the shell is measured tan-tan and not seam-seam. This way, the straight flange is included as part of the shell surface area calc rather than the head. IMO, it's simpler this way and it's less ambiguous.

Using a 90-17 torispherical head as an approximation, I get a volume of 132.7 ft3 (993 gallons) and a surface area of 110 ft3 (compared to 130.6 ft3 and 108.4 ft2 for my previously calculated 2:1 SE head). Using the 90-17 approximation, I get a dish height which is not quite equal to 1/4 the diameter: dish height = CR - SQRT[(KR + (D/2 - KR) - CR)(KR - (D/2 - KR) - CR)] = 0.9 - SQRT[(0.17 + (1/2 - 0.17) - 0.9)(0.17 - (1/2 - 0.17) - 0.9)] = 0.249 < 0.25. This explains the slight differences between the 90-17 head and a true 2:1 SE head.

[ziba]

I have a vessel 5.5 m S/S and Dia=2.24 m, I used formula  AWetted =2LR* COS-1( R-H/R) abased on excel file, it would give you different number from excel file.

 A  wetted= 2x 5.5 x1.2 Cos-1(1.212-2.12/1.22)       My max level of liquid in vessel is H=0.87X2.24=1.94 m, How come I do not get the same surface area, when I calculate it by hand?

[Bobby Strain]

Google my name to find my wesite. Check your answer with software there.

 

Bobby Strain